Skip to main content

1235 - Maximum Profit in Job Scheduling (Hard)

https://leetcode.com/problems/maximum-profit-in-job-scheduling/

Problem Statement​

We have n jobs, where every job is scheduled to be done from startTime[i] to endTime[i], obtaining a profit of profit[i].

You're given the startTime, endTime and profit arrays, return the maximum profit you can take such that there are no two jobs in the subset with overlapping time range.

If you choose a job that ends at time X you will be able to start another job that starts at time X.

Example 1:

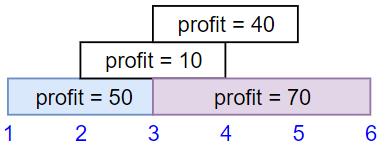
Input: startTime = [1,2,3,3], endTime = [3,4,5,6], profit = [50,10,40,70]
Output: 120
Explanation: The subset chosen is the first and fourth job. 
Time range [1-3]+[3-6] , we get profit of 120 = 50 + 70.

Example 2:

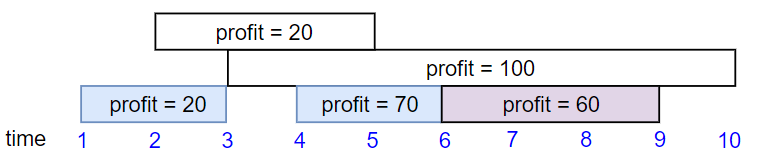
Input: startTime = [1,2,3,4,6], endTime = [3,5,10,6,9], profit = [20,20,100,70,60]
Output: 150
Explanation: The subset chosen is the first, fourth and fifth job. 
Profit obtained 150 = 20 + 70 + 60.

Example 3:

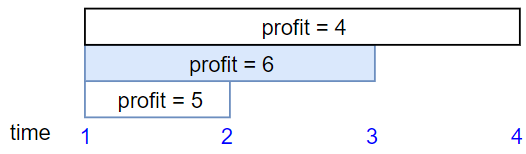
Input: startTime = [1,1,1], endTime = [2,3,4], profit = [5,6,4]
Output: 6

Constraints:

  • 1<=startTime.length==endTime.length==profit.length<=5∗1041 <= startTime.length == endTime.length == profit.length <= 5 * 10^4
  • 1<=startTime[i]<endTime[i]<=1091 <= startTime[i] < endTime[i] <= 10^9
  • 1<=profit[i]<=1041 <= profit[i] <= 10^4

Approach 1: Dynamic Programming​

Written by @wingkwong
// TLE - idx + 1 is too slow
class Solution {
public:
    int jobScheduling(vector<int>& startTime, vector<int>& endTime, vector<int>& profit) {
        int n = startTime.size();
        // rearrange the input
        vector<array<int, 3>> events;
        // put them together
        for (int i = 0; i < n; i++) events.push_back({startTime[i], endTime[i], profit[i]});
        // sort by startTime
        sort(events.begin(), events.end());
        // 1D dp
        vector<int> dp(n, -1);
        function<int(int,int)> dfs = [&](int idx, int end) {
            // reached all events
            if (idx == n) return 0;
            // current start time <= previous end time
            // cannot take it -> move to the next one
            if (events[idx][0] < end) return dfs(idx + 1, end);
            // calculate before -> return immediately
            if (dp[idx] != -1) return dp[idx];
            // so we only have two choices left, we either
            return dp[idx] = max(
                // choice 1: take this job and move to the next one from this end time
                events[idx][2] + dfs(idx + 1, events[idx][1]),
                // choice 2: not take this job and move to the next one
                dfs(idx + 1, end)
            );
        };
        return dfs(0, 0);
    }
};
Written by @wingkwong
// ACCEPTED by utilising lower_bound to move to the next available index
// based on the above solution
class Solution {
public:
    int jobScheduling(vector<int>& startTime, vector<int>& endTime, vector<int>& profit) {
        int n = startTime.size();
        // rearrange the input
        vector<vector<int>> events;
        // put them together
        for (int i = 0; i < n; i++) events.push_back({startTime[i], endTime[i], profit[i]});
        // sort by startTime
        sort(events.begin(), events.end());
        // 1D dp
        vector<int> dp(n, -1);
        function<int(int)> dfs = [&](int idx) {
            // reached all events
            if (idx == n) return 0;
            // calculate before -> return immediately
            if (dp[idx] != -1) return dp[idx];
            vector<int> v = {events[idx][1], 0, 0};
            // search for the next available idx
            int nxt = lower_bound(events.begin(), events.end(), v) - events.begin();
            // so we only have two choices left, we either
            return dp[idx] = max(
                // choice 1: take this job and move to the next one from this next event
                events[idx][2] + dfs(nxt), 
                // choice 2: not take this job and move to the next one
                dfs(idx + 1)
            );
        };
        return dfs(0);
    }
};

Similar Problems​